Batch 3 - Class 233 - Gerrymandering
(zoom)
Pre-Class Exercise
- Read "The Adventures of the Dancing Men", a Sherlock Homes story. Or solve it here www.aamt.edu.au/content/download/18716/.../nmd12_dancing_men.pdf
Attendance Kabir, Vansh, Kushagra, Rhea, Rohan, Anshi, Vivaan, Angad, Shikher, Aashvi, Anishka, Arnav, Arjun, Aarkin, Ayush, Rehaan, Advay, SidharthM
Class puzzles
Destroying Democracy (aka Gerrymandering)
Democracy can be attacked by altering the boundaries of voting regions. Its English word is "gerrymandering". In this puzzle, we are going to be the evil red wizards trying to subvert democracy.
.png)
If there are six wizards, as above, can you demarcate voting regions so that the reds win. The rules
- The largest region can't be 2x or larger than the smallest voting region.
- All regions must be rectangular.
- Each voter must be in exactly one region.
For example the following demarcations fail.
.png)
.png)
.png)
Can you find a winning demarcation?

Now, lets try that for bigger countries.
.png)
.png)
Can you win the above elections with fewer wizards?
.png)
Can you get the 11 wizards to win this 36 people election?
.png)
.png)
Here are our numbers summarized so far. Can you form a hypothesis on minimum number of wizards required for larger elections? 1/4? 1/5? 1/6?
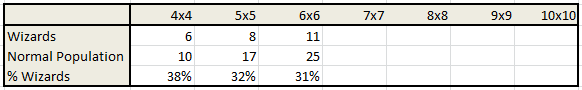
Why don't we try some larger ones.
.png)
.png)
.png)
.png)
.png)
.png)
Our new table looks like
.png)
- Can you now form a hypothesis?
- Reds need to be >50% of “a” regions they win, with x people each (so 0.5xa reds amongst total xa)
- They can be zero in another <a regions, which can each be twice as large as winning regions (so zero amongst total 2xa)
- Combine, 0.5xa out of 3xa, so about 1/6th - you can win elections with 1/6th representation!
Homework:
- Simpson Paradox: When one analyzes the voting records of democrats and republicans during the American Civil Rights Act, 1964, following facts emerge
- In the northern states, 94% of Democrats voted for the act, compared with 85% of Republicans
- In the southern states, 7% of the Democrats voted for the act, compared to 0% of Republicans
- Who showed more support for the act - Democrats or Republicans, as percentage of total Democrat and Republican population respectively.
- Answer: Consider the following:
.png)
- What causes this non-intuitive answer? While there is abysmal voting by republicans in south, there are only 10 of them, so even if one vote had swung, it would look like 10%. On the other hand, there are lot of democrats in South, but very low support.
References: